Mathieu Coquerelle

Contact

Mathieu COQUERELLE
Laboratoire I2M
Université de Bordeaux – bât A11
351 cours de la libération
33405 Talence Cedex
mathieu.coquerelle@bordeaux-inp.fr

Positions

  • Associate Professor at I2M and ENSEIRB-MATMECA, since Sept. 2015:
    • Fluid mechanics and Numerical methods ; specialized in multiphase flows, interfaces dynamic and tracking, level set method, contact line dynamic
    • Project: development of the Notus CFD code
    • Teaching: multiphase flows, numerical methods, CFD Industrial codes, scientific computing
    • Head of the Fluid and Energy specialty of the Matmeca department.
    • Former head of the Fluid Mechanics team of the TREFLE/I2M department
  • Post-doc. at I2M, Apr. 2014 – Aug. 2015:
  • Ph.D. at Laboratoire Jean Kuntzmann, Grenoble, Sept. 2004 – Nov. 2008 
    • Thesis: Fluid-structure interaction computation with vortex methods and applications to image synthesis
    • Keywords: fluid-structure interaction, vortex, level set, image synthesis
    • Supervisors: Georges-Henri Cottet and Marie-Paule Cani
  • Assistant Professor at ENSIMAG and Université Joseph Fourier

Research interests

Projects
  • Ocean waves damping by rain drops
  • Surface tension driven flows and contact line dynamic
Physics
  • Incompressible Navier-Stokes equations
  • Multiphase flow, surface tension, contact line
  • Interfaces (reconstruction, transport, phase change, etc.)
Numerics
  • Level set methods
  • Closest point methods
  • Coupled Eulerian-Lagrangian methods
  • Interpolation and high-order schemes
  • High Performance Computing

Papers (my Research Gate page)

  • Félix Henri, Mathieu Coquerelle, Pierre Lubin. An efficient hybrid advection scheme in a level set framework coupling WENO5 and HOUC5 schemes based on kink detection. Under revision. (HAL version)
  • Deewakar Sharma, Mathieu Coquerelle, Arnaud Erriguible, Sakir Amiroudine. Adaptive interface thickness based mobility—Phase-field method for incompressible fluids. International Journal of Multiphase Flow, Volume 142, 2021, 103687. (DOI)
  • Félix Henri, Mathieu Coquerelle, Pierre Lubin. Geometrical level set reinitialization using closest points method and kink detection for thin filaments, topology changes and two-phase flows. Journal of Computational Physics Volume 448, 1 January 2022 (DOI, HAL version)
  • Florian Desmons, Mathieu Coquerelle. A generalized high-order momentum preserving (HOMP) method in the one-fluid model for incompressible two phase flows with high density ratio. Journal of Computational Physics, 2021, 110322. (DOI, HAL version)
  • Thanh – Nhan Le, Mathieu Coquerelle, Stéphane Glockner. Numerical simulation of moving contact line in wetting phenomena using the Generalized Navier Boundary Condition. Congrès Français de Mécanique (CFM) 2019. (pdf)
  • Mathieu Coquerelle, Stéphane Glockner. A fourth-order accurate curvature computation in a level set framework for two-phase flows subjected to surface tension forces. Journal of Computational Physics, 2016, vol. 305, p. 838-876. (DOI, HAL version)
  • Mathieu Coquerelle, Georges-Henri Cottet. A vortex level set method for the two-way coupling of an incompressible fluid with colliding rigid bodies. Journal of Computational Physics, 2008, vol. 227, no 21, p. 9121-9137. (DOI, HAL version)
  • Georges-Henri Cottet, Guillaume Balarac, Mathieu Coquerelle. Subgrid particle resolution for the turbulent transport of a passive scalar. Advances in Turbulence XII, 2009, p. 779-782.
  • Mathieu Coquerelle, Jérémie Allard, Georges-Henri Cottet, Marie-Paule Cani. A vortex method for bi-phasic fluids interacting with rigid bodies. arXiv preprint math/0607597, 2006.
  • Mathieu Coquerelle. Thèse de doctorat : Calcul d’interaction fluide-structure par méthode vortex et application à la synthèse d’images, sous la direction de M.-P. Cani et G.-H. Cottet. Soutenue le 25 septembre 2008 à l’INP Grenoble. (HAL version)

Ph.D. students

  • Félix HENRI (2018-2021): Numerical methods for the simulation of rain drops on ocean waves. (co-guidance with P. Lubin, I2M) (HAL version, in French)
  • Thahn Nhan LE (2016-2019): Modeling of the triple contact line (between air, water and a solid) (co-guidance with S. Glockner, I2M)

Internship tutoring

  • Icham CHERBLANC: Master 2 thesis (2021): Improving the Level Set Methods with Maximum Likelihood approaches (co-guided with Annabelle Collin).
  • Thomas AGOSTINI: Master 1 thesis (2021): Modeling and fast computation of bubble rise in glasses.
  • Clément BENAZET: Master 1 thesis (2020): Improving the Closest Point algorithm for Level Set methods.
  • Lola ROCAMORA: Master 1 thesis (2019): Study of the free fall of a rain drop until terminal velocity using Notus CFD.
  • Patrice SEBASTIANO: Master 1 thesis (2018): Experimenting the epsilon-level set approach for multi-phase flows simulation in Notus CFD.
  • Maxime LARGEAUD: Master 1 thesis (2018): Implementation and enhancement of high-order interpolation with Hermite and WENO schemes.
  • Nicolas GODINAUD: Master 1 thesis (2018): Implementation of the Particle Level Set method for Notus CFD.
  • Florian DESMONS: Master 2 thesis (2017): Understanding, modeling and simulation of the impact of a rain drop on a water wave
  • Guillaume DUMAS: Master 1 thesis (2016): Modeling and simulation of the impact of a rain drop on a flat surface

Involvement in Notus CFD

  • Specific code development
    • Interface advection with level set, phase field and front-tracking methods
    • Explicit inertial term for the Navier-Stokes equation
    • Continuum Surface Force for surface tension forces
    • Closest-point method for curvature computation
    • Finite Volume and Finite Difference schemes
    • Interpolation and extrapolation with high-order schemes (Lagrange and WENO)
  • Verification and validation
    • ​Surface tension
    • Rayleigh-Taylor instabilities
    • Free surface waves
    • Bubble rise
    • Water drop(s) fall and impact

Gallery

Applications
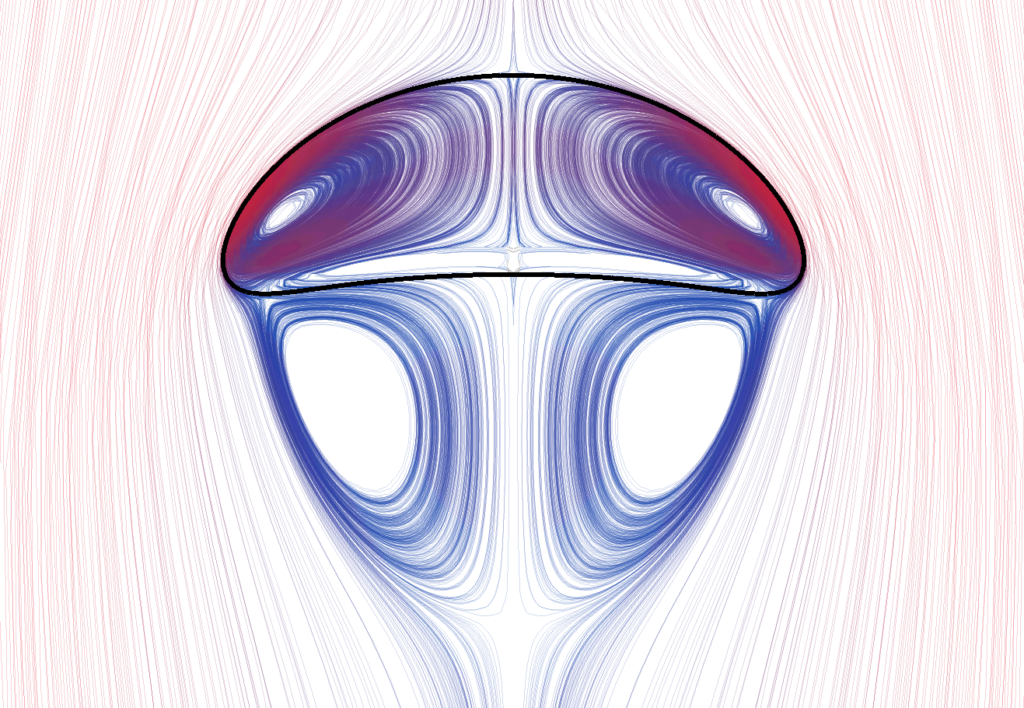
Level Set Reinitialization using Closest-Points (as presented in DOI)
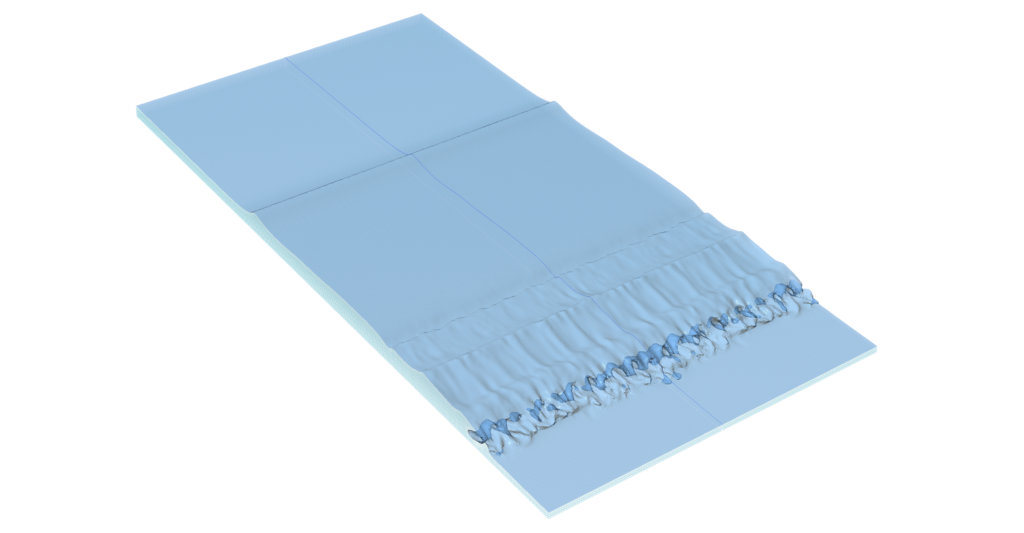
3D dam break test case (as presented in DOI)
Impact of a droplet on a liquid pool – Cole III experiment (as presented in DOI)

Axisymmetric simulation, ~ 2M cells, using high-order methods.